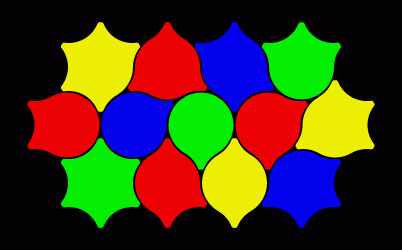
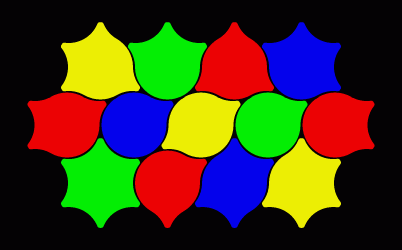
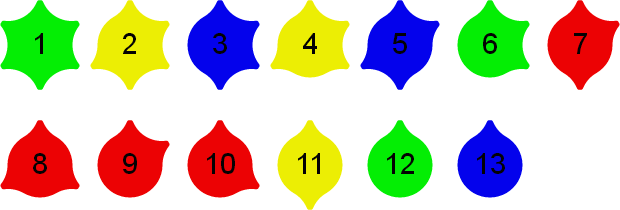
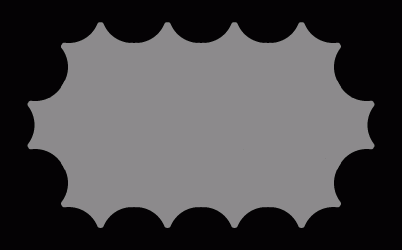
Kwazy Quilt is a two-dimensional packing puzzle consisting of 13 pieces that need to be placed within a frame. Imagine packing circles of the same size together as closely as possible. This gives you a hexagonal arrangement, where each circle is surrounded by six other circles, and with six small triangular holes between them. The pieces of this puzzle consist of a circle with one or more of those curved triangular shapes added. Every possible shape occurs once, except that the circle with one added triangle occurs twice. The frame into which the pieces are to be placed has an empty area consisting of a row of 5 circles with rows of 4 circles nestled into it on both sides, together with all the triangular areas adjacent to those circles. The pieces and the frame are shown below.
The pieces come in four different colours. As an extra challenge, you can solve the puzzle such that no two pieces of the same colour are adjacent.
This puzzle was made by Kohner Brothers in 1960s and 1970s. Early versions have a frame made of cardboard, but later versions (like mine from 1972) have a plastic frame. The colour of the pieces differs between versions, though they do all use the same choice of identically coloured pieces.
The other picture above is of a Soviet version of the puzzle, made in Donetsk, Ukraine. It is smaller, and all the pieces have the same colour. The leaflet claims it has 1640 solutions, but I believe this to be incorrect. The text on the leaflet translates roughly to the following:
Ministry of Light Industry USSR
Donetsk toy plant
340037, Donetsk - 37, Kirov str. 90
PUZZLE GAME
This puzzle game belongs to the combinatorial games, many of which became the subject of great interest and enthusiasm.
Addressing the challenges posed by these games has been one of the branches of mathematics - combinatorics, which examines some of the operations over a certain number of items - letters, numbers, and geometric shapes. etc.
The proposed puzzle is to pack the tiles in the frame box. But the figure shown is not the only way to place them. There are 1640 solutions. Apply your resourcefulness in searching them. Tiles may be laid down on either side.
It contributes to the development of logical thinking, spatial imagination, ability to concentrate, and persistence in achieving your goal.
Set contents:
- tiles of various shapes - 13 pieces
- packing box - 1 pce
- leaflet - 1 pce
For children from 7 to 15 years
Treatment type: washing
Article: U-085-2749
Price 0 rub. 45 kop.
OCT 17-296-75
Price List 085-02-1980 art. 156
This puzzle has also been produced by Tenyo in Japan in their "Beat the Computer" series of puzzles.
The pieces fill up the frame without any holes. From the shape of the frame it is then immediately clear that the pieces at either end of every row must be a shape with two adjacent triangular points. The two middle locations of an outer row each have one triangular point on the outside, but also have one triangle in between them that one of the pieces must fill. Therefore one of the two pieces in the middle two locations of an outer row also needs to have two adjacent triangular points. All together we need 8 such pieces, and there happen to be exactly 8 of them in the set.
This all greatly restricts the number of possibilities. First fill in the leftmost location on each row. Consider the triangle location that lies on the top edge, between the first and second circle of the top row, and suppose that location is not filled by a point from the piece you placed at the left of the top row. It will then have to be filled by the piece you place next to it. That piece therefore has two adjacent points on it. We already know then that the third piece on the top row does not have adjacent points, so the second piece will also have to fill the triangle between it and the third piece. This means that the second piece will have (at least) three adjacent points in it.
These considerations lead to the following solving strategy:
I have run a computer search to find all solutions. There are 96 solutions with the star shaped piece (piece 1) at the left end of the middle row (mirror images excluded), and 1701 with the star shape at the left end of the bottom row. Apart from rotations and mirror images of these 1797 arrangements, there are no other solutions.
There are only two solutions in which no two pieces of the same colour are adjacent. These two solutions are shown below.