|
|
 |
 |
|
Isohedral Tilings
and
their Templates
Introduction
Isohedral tilings have two defining characteristics; they consist of only one shape of tile, and they have translation symmetry. The single tile, depending on its detailed shape, can appear in the same orientation throughout the whole tiling or more usually in a number of different rotated or reflected orientations which fit together. The translation symmetry demands that any aspect of the tiling, be it a single tile in a particular orientation or a group of tiles, repeats itself periodically over the whole tiling.
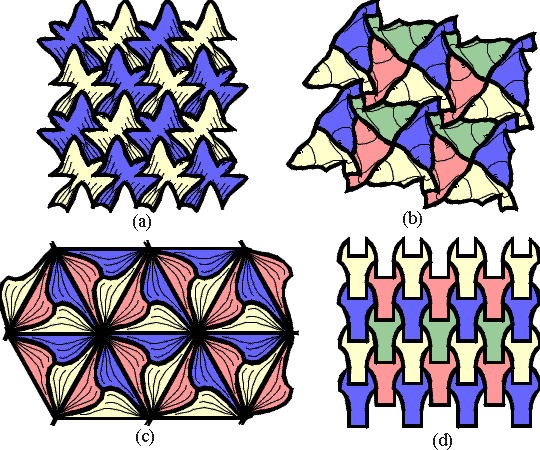
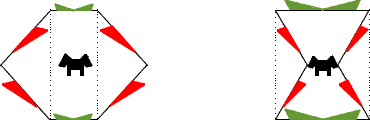
Each of the above sections of infinite tilings consists of a single tile shape.In (a), the bird-shaped tile appears in two different orientations, one a reflection of the other, in alternate rows; in (b), the fish appears in four different orientations, rotated at right angles to one another; in (c), the tile appears in six different orientations, both rotated and reflected from one another; in (d), the spanner appears in the same orientation throughout. In each case it is clear how the pattern, based on a single tile shape, will repeat itself periodically over a complete tiling. Each tiling is therefore isohedral.
In these and all other tilings which will be shown, the different tile colours are of no relevance. They are used only to allow adjacent tiles easily to be distinguished from one another. The minimum number of colours required to achieve this is always used. This follows a convention adopted by Escher
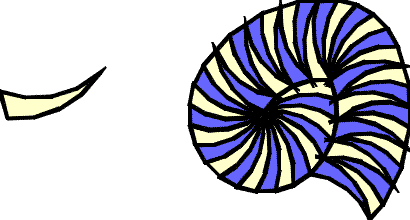
A single shape which can fit together with itself need not do so in a periodic fashion. The tiling formed is therefore not isohedral. It is called monohedral. The following example shows a tile and a section of such a monohedral tiling formed with it. It is fairly clear that extending the spiral-type pattern to complete the whole tiling will not produce any periodically repeating pattern.
The tilings (a) and (b) are based on tile shapes originally designed by Escher. He produced almost a hundred different shapes which fit together in a variety of ways with themselves to produce isohedral tilings. They are all shapes that by appropriate decoration can be made to resemble animate objects like fish, birds, reptiles, etc. Many more examples of shapes which tile isohedrally can be found in Islamic art. They are all of a geometric nature, and examples will be seen later.
With so many examples available, it is natural to seek answers to the following questions. How can each different type of isohedral tiling be characterised? Can the total number be enumerated and if so, how many are there? Grunbaum and Shephard (G&S) in their book ‘Tilings and Patterns’ (Freeman,New York, 1990) provided the answers. They developed a useful way of characterising each type of tiling and used that characterisation to enumerate all the types of isohedral tilings. They found that there is a total of 93 types. Their characterisation of each type of tiling is essentially based on one of two related concepts -- the so-called incidence symbol of the tiling or the tile's adjacency diagram. However neither of these is always sufficient to remove ambiguities, and the topological type of the tiling is also required for a unique characterisation. The incidence symbol is particularly useful in the enumeration process. No such enumeration of monohedral tilings has yet been achieved.
An alternative way of characterising the 93 isohedral tilings is offered here using the concept of tile templates. A template is sufficient to characterise each type of tiling type uniquely, and to do this in a visually informative way. We shall descibe this alternative approach in some detail.
The Concept of Templates
A template is a skeleton from which can be formed all the many tile shapes which fit together with themselves in the same way, to form tilings of the same type. It defines uniquely the tiling type. To understand this in more detail, it is useful to work backwards from a number of tilings. Let us examine the tilings (a) - (d) in turn. This will bring out all the relevant aspects of the concept of templates.
First of all take a small section of the tiling (a) which shows one central cream-coloured tile and all those adjacent to it, ie all the blue tiles with edges in common with it. The vertices of this tile, ie those points where
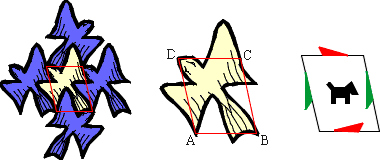
it meets at least two other tiles, are joined by straight lines as shown;the quadrilateral formed with its vertices labelled A to D is also shown separately along with the single cream tile . From the tiling sample we can see that the two sides of the bird fit together so that the lines AD and BC must be equal in length and the tile edges from A to D and from B to C must be the same shape. They are therefore marked on the final drawing of the quadrilateral with the same green half arrowheads. The half arrowheads indicate three things - sides with the same colour of half arrowhead must have the same shape, ie the colour labels the shape, the direction of the half arrowhead gives a direction to the shape, and using only a half rather than a full arrowhead gives a necessary left or right handedness to the shape. The two sides of the template can be shaped in any fashion, and as long as these rules associated with the half arrowheads are followed the shaped tiles so-formed will fit together side by side as the birds do. We can also see from the way the top and bottom of the birds fit together that the lines AB and DC must be parallel and of the same length and that the shapes of the tile edges along the top of the tile between D and C and the bottom between B and A are also the same. But here one is obtained from the other by reflection in a vertical axis - hence the relative orientation of the two red half arrowheads. The shapes are the same so the arrowheads are both red, but left-to-right in one is the opposite in the other, as is the handedness of the two. Also since AD and BC are equal and parallel and AB and DC are also equal, it follows that ABCD must be a parallelogram. Finally we note that the bird-shaped tile has no symmetries of its own, ie it cannot be rotated or reflected in any way that leaves it otherwise unchanged. This lack of symmetry is retained in the marked parallelogram. To emphasise and make this readily clear, the asymmetric dog is placed inside the it. This marked parallelogram forms the template for the bird tiling.
This template example is already more general than the bird shape, since its sides can be shaped in any way we choose as long as the shapes obey the constraints implied by the arrowheads. The sides can obviously be shaped to form the bird, but they can be shaped in an infinitely large number of other ways which still produce an overall shape which forms a tiling in the same way as the birds.
The arrowhead markings on the template have been introduced to define how associated tile edges can be shaped. But they serve another purpose. The template itself can be regarded as a tile, with the markings determining how it can be fitted together with itself to form a tiling with all the same basic properties as that formed by the bird shapes. The template edges must be fitted together so that the arrowheads coincide, as shown. This also shows how the asymmetric dog clearly indicates the relative orientation of the various template tiles.

Finally, although we have arrived at a particular parallelogram as the template for the bird shape with which we started, none of the constraints on this parallelogram limit us to it. It is not difficult to realise that any parallelogram with the same shaping and fitting constraints on its sides also forms a template which generates a tiling with all the same properties. Thus we can characterise this type of tiling by a template which is any parallelogram whose sides can be shaped as indicated by the half-arrows.
Let us now turn our attention to tiling (b), and as before examine a small section consisting of one tile and its neighbours, ie its adjacents plus all the other tiles which meet at its vertices. Joining the vertices of this tile with straight lines gives a pentagon. Notice first of all from the tiling section that at the vertex C, the same vertex of four different tiles meet, forcing the pentagon angle at C to be 90°. The same applies to the angle at E. Also we see from the tiling that the shapes joining B to C and D to C must be the same, one being obtained from the other by rotating the shape by 90° about C. Therefore BC must equal DC and they must be marked in the template with the same colour,
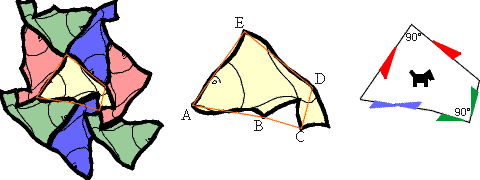
green, of half-arrow, arranged so that one is obtained from the other by 90° rotation, as shown. The same discussion also applies to the angle at E and the shapes joining A to E and D to E, and leads to the red half-arrow markings on the template.
The tile edge which joins A to B is different. From the tiling we see that it fits on to itself rotated through 180°, ie A fits to B and vice versa. The shape along one half of this edge must therefore be obtained from that along the other by rotating it through 180° about the mid-point. This is indicated in the template by the double half-arrows. The edge is not paired with any other and so its arrowheads have their own unique colour. A 180° rotation is equivalent to two reflections in orthogonal axes through the centre of rotation, and on this basis the edge joining A and B is said to have central reflection symmetry.
As in the previous example, the template has no internal symmetry of its own and this is indicated again by the asymmetric dog.
The only constraints we have encountered on the form of the pentagon is that AE and ED are orthogonal and equal to one another, as are BC and CD. In fact any pentagon with two pairs of adjacent sides, such that within each pair the sides are orthogonal and equal, forms a template, when its sides are appropriately marked, for tilings of the type exemplified by our fish. We can see this by building a tiling from a pentagon with different proportions.
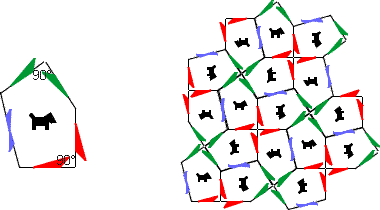
Some other aspects of templates are revealed by examining our tiling (c). Again we look at a small section containing one tile and all its neighbours and see that joining its vertices with straight lines this time gives a triangle. Notice first in the tiling that the vertex C meets itself in two other tiles indicating that the angle at C must be 120° as shown 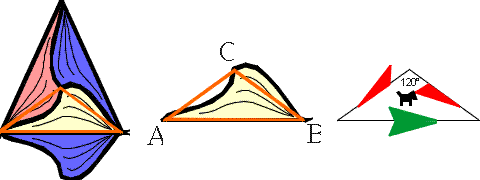
in the template. Also the sides AC and BC fit together and must therefore be equal and be marked as shown in the template. The side AB is different to anything we have met before. In the tiling, two tiles meet along this side in such a way that one is the mirror reflection of the other in the side. This can occur only if AB is straight. It cannot be shaped. To indicate this we mark it in a colour unique to it with a full arrowhead. The colour must be unique since it fits on to itself and no other side is involved. A full arrowhead is used since we require a mark which will fit on to itself only in reflection in the side and precludes any possibility of placing together two versions of the tile rotated by 180° relative to one another. Again the template has no internal symmetry of its own and this is indicated again by the asymmetric dog
In this example the only geometric constraints placed on the template are that AC and BC should be equal and at an angle of 120°. Thus a template for the type of tiling exemplified here is any 120° isosceles triangle. Unlike our previous two examples, this template has a fixed shape and only its size is arbitrary. The template tiling is therefore very similar to our example.
Finally let us examine a small section of tiling (d), again with one tile surrounded by all its neighbours. The tile has six vertices so that the
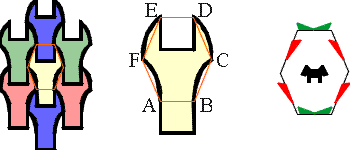
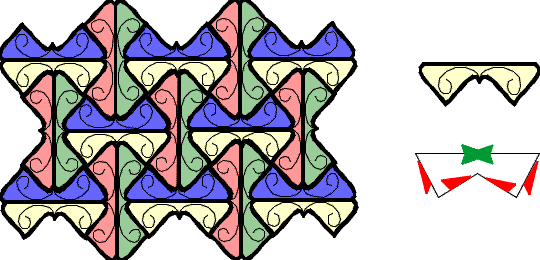
template is a hexagon. The sides AF and CD fit together as do BC and FE. Also in contrast to our previous examples, the tile in this case is symmetrical; it is unchanged by reflection about an axis running centrally up and down it. Thus these four sides of the template must all be equal and they can all be shaped but only in similar fashions as dictated by the red half-arrowheads. Also, although the shapes between A and B and between E and D fit together, they must be shaped symmetrically to maintain the required symmetry. Thus AB and DE must be equal and marked with double half-arrowheads in the template to indicate their symmetry To emphasise the tile symmetry the template is marked with the symmetric dog pattern.
The only geometrical constraints on the template are that AB and ED are equal and parallel, as are AF and CD and also BC and EF, with the latter four all being equal to one another. Thus more generally, the template is any hexagon formed by mounting two identical isosceles triangles on to opposite sides of a rectangle so that they are mirror images of one another. This can be achieved in two different ways as shown with the basic rectangle indicated by the dotted line.
A template tiling based on the form of the right hand template is also shown.
Returning to the type of tiling we saw in (c) where the shaping of one side of the template was not allowed and this was indicated by a full arrowhead marking. There are types of tilings where this marking is inappropriate due to the symmetry of the tile. The tiling shown below is constructed from a tile which has one unshaped edge and also has reflection about an axis orthogonal to this straight edge. Thus a tile joined to another by this edge is related to it either by a mirror or a central reflection in the edge; they are equivalent. In such cases the additional central reflection symmetry is indicted in the template by a full double arrowhead rather than a single one, as shown.
|
 |
 |
|
Summary of Templates
|
 |
 |
|
Having now seen what templates are all about, we shall summarise their properties here.
|
|
|
 |
 |
|
Each of the 93 isohedral tilings, labelled IH1 to IH93 by G&S, is uniquely defined by its template.
Templates take the form of triangles, quadrilaterals, pentagons, and hexagons, with geometric constraints.
All the sides of templates are marked by coloured half or full arrowheads. These serve two purposes. They determine how the template or any tile formed from it fit together to form a tiling; template edges must be placed together so that markings of the same form and colour overlap. They also show how the template sides can be deformed to form shapes that will tile in the same way as the template; sides with markings of the same form and colour must be deformed in the same way relative to the mark.
There four types of markings.
|
|
|
 |
 |
 |
 |
 |
 |
|
This is the commonest. There must be at least two and always an even number of sides marked in this way in the same colour. The side can be shaped in any arbitrary fashion.
|
|
|
|
This marking labels a side that must be fitted on to itself using a 180° rotation of the template, or to any other side similarly marked in the same colour. Any deformation of the side must have a central reflection.
|
|
|
|
This marking forces any deformation of the side to be symmetrical with respect to a reflection in an axis through the midle of the side and orthogonal to it.
|
|
|
|
These markings indicate that the side cannot be deformed. The single arrowhead indicates that the side must be fitted to itself by a mirror reflection of the template or tile; the double arrowhead indicates that fitting can be by mirror or central reflection.
|
|
 |
 |
|
In many cases the angle beween certain sides of a template is fixed at either 60°, 90°, or 120°. Sometimes this is obvious when the template, for example, is defined to be a rectangle or equilateral triangle. However it is not always obvious and in cases of any doubt the size of a fixed angle is marked on the template.
|
 |
 |
|
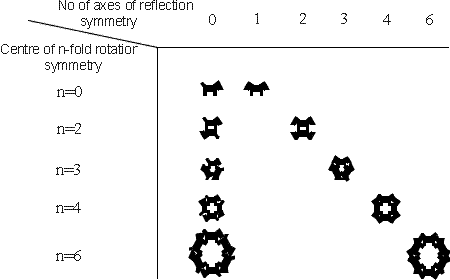
Templates can be completely asymmetric or have internal rotation symmetry about their centres or reflection symmetry about axes through their centres. These symmetries are shown by the arrowhead markings on the sides of the templates, but it is very useful to show the symmetry more explicitly by a suitable symbol at the centre of the template. The dog patterns shown below do this very well, clearly showing an asymmetric pattern as well as patterns having two-, three-, four-, and six-fold rotation, with or without axes of reflection symmetry.
 |
|
 |
|
Classification of Tilings
There are 93 types of isohedral tilings which Grunbaum and Shephard classified according to their so-called topological type. For consistency their classification and labelling are used here.
The concept of topological type arises as follows. The vertices of a tile in a tiling are those points on its perimeter at which it meets at least two other tiles. Tiles which form isohedral tilings have three, four, five, or six vertices corresponding to their templates being triangles, quadrilaterals, pentagons, or hexagons. Each vertex can be characterised by its valence, this being the number of tiles which come together at the vertex when a tiling is formed. The topological type of the tiling is defined by the valences of the vertices of its tile enumerated in order round the tile perimeter.
Our tilings (a) - (d) can be used to illustrate the concept. In (a), the bird has four vertices at each of which four tiles meet, and the topological type of the tiling is therefore 4.4.4.4, usually abbreviated to 44. In (b), starting at the fish's head and moving around by its underside, we meet five vertices of valences 3, 3, 4, 3, 4 and so the topological type is 32.4.3.4. In the same way, the topological types of the tilings (c) and (d) are respectively 3.122, and 36.
The 93 different types of isohedral tilings belong to 11 different topological types. Grunbaum and Shephard gave each tiling an IH number allocated according to the following table
Topological type Template type IH numbers
36 Hexagon 1 - 20
34.6 Pentagon 21
33.42 Pentagon 22-26
32.4.3.4 Pentagon 27-29
3.4.6.4 Quadrilateral 30-32
3.6.3.6 Quadrilateral 33-37
3.122 Triangle 38-40
44 Quadrilateral 41- 76
4.6.12 Triangle 77
4.82 Triangle 78-82
63 Triangle 83-93
|
 |
|
Symmetry of Tilings
As well as an individual tile having some internal symmetry which we indicate with a dog pattern, the tiling formed by the tile has its own individual symmetries. The tiling symmetries belong to one of 17 so-called crystallographic groups. The 17 groups all contain the translations which leave the tiling unchanged, defined by a set of vectors T(m,n) = ma + nb where m and n are any positive or negative integers or zero, and a and b are two non-parallel vectors characteristic of the tiling and referred to as its basic translations. In addition, the symmetry groups contain a number of rotations and/or reflections which also leave the tiling unchanged. The rotations can be through 1800, 1200, 900, or 600 about certain centres referred to respective as centres of two-, three-, four-, and six-fold symmetry. They are indicated as follows:
|
|
|
 |
 |
|
Centre of two-fold symmetry
Centre of three-fold symmetry
Centre of four-fold symmetry
Centre of six-fold symmetry
|
|
 |
|
The reflections are of two types, mirror and glide. A mirror reflection reflects the tiling in a so-called axis of reflection. If the tiling is unchanged by this reflection the axis is one of mirror reflection symmetry and is indicated thus:
|
 |
|
A glide reflection involves not only reflection in an azis but also a movement or glide by a specific amount along that same axis. If the tiling is unchanged under such an operation, the axis is one of glide reflection symmery and is indicated thus:
|
 |
|
The following table shows the (less than obvious) labels of the 17 crystallographic groups and the numbers of different types of centres of rotation and axes of reflection symmetry which each contains:
|
 |
|
Thus, for example, the group p31m contains two centres of three-fold rotation symmetry, 1 axis of glide reflection symmetry and 1 of mirror reflection symmetry.
In some types of tilings, eg those with p1 symmetry, the tile appears in only one orientation, but in others it takes up a number of different orientations, ie rotated or reflected, associated with the overall symmetry of the tiling; the orientations are determind by the markings on the sides of the tile's template. It is useful and always possible to construct a cluster of adjacent tiles, containing one, and only one tile in each of the possible orientations, so that the whole tiling is formed by this so-called translation cluster being repeated at positions determined by the translation vectors T(m,n). When the tile appears in only one orientation in the tiling the translation cluster consists of a single tile, but more frequently a cluster consists of two or more tiles. A multi-tile cluster is not unique as the differently oriented tiles required to form it can be placed adjacent to one another in a number of ways.
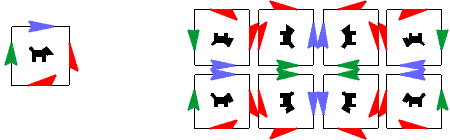
As an example, for the tiling of labelled IH56, its template is the following square which appears in the tiling in eight different orientations which can fit together as shown to form a translation cluster as follows
|
 |
|
|
 |
 |
|
A section of the tiling formed is also shown below with the translation cluster and the basic translation vectors indicated by the heavy outlining. The tiling belongs to the symmetry group p4g and also shown are examples of the centres of 2- and 4-fold rotation symmetry, the two axes of glide reflection symmetry, and the one of mirror reflection symmetry which it possesses. All these are replicated periodically throughout the tiling according to its translation symmetry.
|
 |
|
|
 |
 |
|
Decoration of Tiles
Of the 93 different isohedral tilings, 50 are characterised by templates, all of whose sides can be shaped; the remaining 43 have templates with at least one side which cannot be shaped, and of these 21 cannot have any of their sides shaped at all.
Shaping the sides of a template produces a tile with the correct symmetry; the tile may or may not be decorated internally, but if it is, its symmetry must be preserved by ensuring that no internal decoration has a lower symmetry.
The situation is different if a template’s sides are not shaped in forming a tile, either by choosing not to shape all the sides that can be, or in the case of the 21 tilings where no shaping of any side is allowed. The undecorated tile formed can then have a symmetry higher than it should; this must be reduced by decorating the tile internally to reduce its symmetry, so that the symmetry of the tiling formed from it is correct.
The situation is well illustrated by the five templates of the 93 which are equilateral triangles. Only IH88 and IH90 have
|
 |
|
sides which can be shaped. If they are not, IH88 must be given an asymmetric decoration and IH90 a decoration with three-fold rotational symmetry, as indicated by the dog patterns. Of the other four templates, tiles formed from the three, IH87, 89, and 92 must be given internal decoration with the same symmetry as the dog patterns, otherwise their symetry would be too high. However, IH93 is different. Its tile can be given a decoration with the dog pattern symmetry but since that is the same as the equilateral triangle tile shape itself, it is not necessary.
The colouring of tiles can also be considered as another form of decoration, with individual tiles being given different colours. Such decoration does not affect the symmetry of an individual tile. But it can affect the symmetry of the tiling pattern formed by differently coloured tiles if the different colours are taken into account. The symmetries of tilings with different colouring schemes is a subject in its own right which is discussed in detail by Grunbaum and Shephard in their book referred to earlier. As pointed out in the Introduction, we ignore the matter here. The colouring of tiles is used in our tilings simply as a way of distinguishing between different tiles, but ignored in assessing the symmetry of the tiling. Following Escher, we use the minimum number of colours in any tiling that ensures that no tile is adjacent to one of the same colour.
|
|